Three‑phase‑lags thermoelastic infinite medium model with a spherical cavity via memory-dependent derivatives
Downloads
Published
DOI:
https://doi.org/10.58414/SCIENTIFICTEMPER.2024.15.1.21Keywords:
Thermoelastic, three-phase-lags, memory-dependent derivative, fractional calculusDimensions Badge
Issue
Section
License
Copyright (c) 2024 The Scientific Temper

This work is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License.
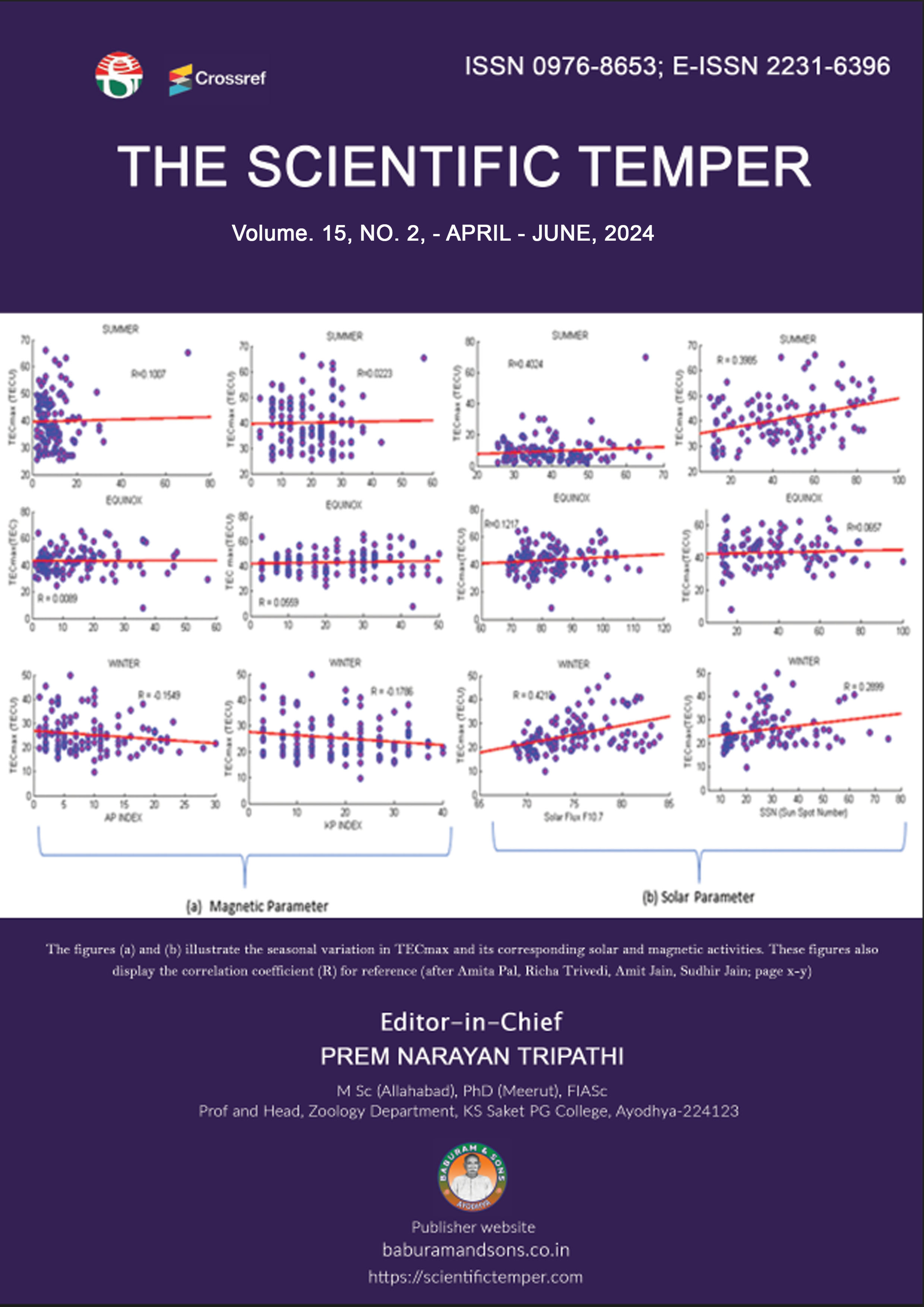
The present study examines the impact of a three-phase lags thermoelastic infinite medium with a spherical cavity subjected to thermal shock in the temperature of its internal boundary. In this study, a new time-fractional three-phase-lag thermoelasticity model with memory-dependent derivatives is utilized. From the suggested model, we recover certain previous thermoelasticity models as special instances. Laplace transform techniques are used. The solution to the problem in the transformed domain is obtained by using the Gaver-Stehfest algorithm. The validity of the proposed theory is evaluated through a comparison with the existing literature. The numerical computations are conducted and represented graphically. The numerical values of field variables show significant differences for a specific material, highlighting important points related to the prediction of the new model. The article’s physical viewpoints could be helpful in the development of novel materials.Abstract
How to Cite
Downloads
Most read articles by the same author(s)
- Ravi Chaware, Sajid Anwar, Sunil Prayagi, Thermoelastic response of a finite thick annular disc with radiation-type conditions via time fractional-order effects , The Scientific Temper: Vol. 15 No. 02 (2024): The Scientific Temper
- Shahala Sheikh, Lalsingh Khalsa, Nitin Chandel, Vinod Varghese, Hygrothermoelastic large deflection behaviour in a thin circular plate with non-Fourier and non-Fick law , The Scientific Temper: Vol. 15 No. 02 (2024): The Scientific Temper